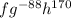The expression is given as,
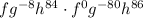
Consider the law of exponents,

Use the above law to simplify the given expression.
Take the terms with same bases together,
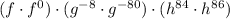
Apply the law,

Thus, the simplified form of the given expression (without any denominator is obtained as,