Question

Brain is rowing across the river but doesn’t know the distance. He looks directly across and sees a pier. Then he walks downstream 400 ft and looks at the pier again. It is now at an 82 degree angle. What is the distance across the river?

Answer 1

From the statement of the problem, we know that:

• Brian looks directly across and sees a pier,

,

• he walks downstream 400 ft, and looks at the pier again,

,

• he is now at an angle θ = 82°.

Using the data of the problem, we make the following diagram:

Where x is the distance across the river.

The diagram constitutes a triangle of:

• angle ,θ = 82°,,

,

• opposite side ,OC = 400 ft,,

,

• adjacent side ,AC = x,.

From trigonometry, we have the following trigonometric relation:

`\tan \theta=(OC)/(AC)\text{.}`

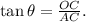
Replacing the data above in the last equation, we have:

`\tan (82^(\circ))=(400ft)/(x).`

Solving for x the last equation, we find that:

`\begin{gathered} x\cdot\tan (82^(\circ))=400ft, \\ x=(400ft)/(\tan(82^(\circ))), \\ x\cong56.21633ft\cong56ft\text{.} \end{gathered}`

Answer

The distance across the river is approximately 56 ft.