We are given the following expression:

The first term is 1 elevated to an exponent. Let's remember that 1 to any exponent is always 12, therefore, we have:
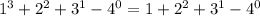
Now we solve the second term which is 2 multiply by itself, therefore, we get:
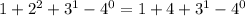
The third term is 3 elevated to 1. Let's remember that any number elevated to 1 is the same number, therefore, we get:

The fourth term is 4 elevated to zero. Let's remember that any number elevated to zero is 1, therefore, we have:
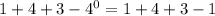
Solving the operations we get:

Therefore, the expression simplifies to 7.