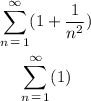Let the sequence a_n be equal to:
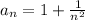
And the sequence b_n be equal to:

Notice that both series diverge:
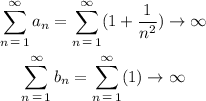
Nevertheless, notice that the sequence a_n-b_n is given by:
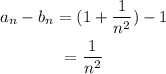
Then:

It is a famous result that:

Then we found two divergent series so that each series diverge and the series of the difference a_n-b_n converge. The series are: