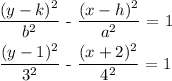ANSWER
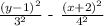
Explanation:
Given information
Center . (-2, 1)
One focus. (-2, 6)
One vertex. (-2, 4)
Recall that, the standard form of a hyperbola function is given below as

where (h,k) is the center of the circle
The next process is to determine the value of h and k
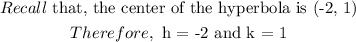
The next step is to determine the value of b by finding the distance between the center and the vertex
![\begin{gathered} \text{Center = (-2, 1) and vertex = (-2, 4)} \\ \text{Distance = }\sqrt[]{(x1-x2)^2+(y1-y2)^2} \\ \text{From the given points, let x1 = -2, y1 = 1, x2 = -2 and y2 = 4} \\ Recall\text{ that, the distance betwe}en\text{ the center and vertex = b} \\ b\text{ = }\sqrt[]{(-2+2)^2+(1-4)^2} \\ b\text{ = }\sqrt[]{0^2+(-3)^2} \\ b\text{ = }\sqrt[]{0\text{ + 9}} \\ b\text{ = }\sqrt[]{9} \\ b\text{ = 3} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/l4f5up9ws4dqofsuwky9ljga9ukprqvs6z.png)
The next step is to find the value of a using the below formula
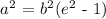
To get the value of a, we need to get the value of e by finding the distance between the center and the focus
Let the distance between the center and the focus be CF
![\begin{gathered} \text{Center = (-2, 1) and focus = (-2, 6)} \\ \text{Distance = }\sqrt[]{(x1-x2)^2+(y1-y2)^2} \\ \text{From the given points, let x1 = -2, y1 = 1, x2 = -2 and y2 = 6} \\ CF\text{ = }\sqrt[]{(-2+2)^2+(1-6)^2} \\ \text{CF = }\sqrt[]{0^2+(-5)^2} \\ \text{CF = }\sqrt[]{0\text{ + 25}} \\ \text{CF= }\sqrt[]{25} \\ \text{CF= 5} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/a8rec4rb6qa3g7xvxrplpz4y7llyur4wif.png)
Recall that, CF = be
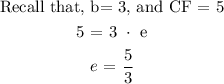
The next process is to determine the value of a from the below formula
![\begin{gathered} a^2=b^2(e^2\text{ - 1)} \\ a^2=3^2\text{ \lbrack(}(5)/(3))^2\text{ - 1)\rbrack} \\ a^2=3^2\text{ ( }(25)/(9)\text{ - 1)} \\ a^2=3^2\text{ (}\frac{25\text{ - 9}}{9}) \\ a^2\text{ = 9 (}(16)/(9)) \\ a^2\text{ = 16} \\ \text{Take the square roots of both sides} \\ \sqrt[]{a^2}\text{ = }\sqrt[]{16} \\ a\text{ = 4} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/1inse1c01f6urkr1a1cygp4yqz9edivs8f.png)
From the overall calculation, we were able to get the following values
h = -2
k = 1
b = 3
a = 4
The next process is to substitute the above value into the hyperbola formula