Question

a merry go round at an amusement park makes 3 revolutions per minute. if the linear speed of a person riding on an outside horse is 3.8ft./sec, how far, in feet is the horse from the center of the merry go round? use 3.14 for pi and round to the nearest tenth.

Answer 1

Givens.

• The angular speed is 3 revolutions per minute.

,

• The linear speed is 3.8 ft/sec.

Use the formula that includes linear speed, angular speed, and radius.

`\omega=(v)/(r)\to r=(v)/(\omega)`

But, we need to transform the angular speed from revolutions per minute to radians per second.

`\omega=3\cdot\frac{\text{rev}}{\min}\cdot\frac{2\pi\text{rad}}{1\text{rev}}\cdot(1\min)/(60\sec)=0.314\cdot\frac{\text{rad}}{\sec }`

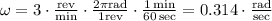
Once you have the right units, find the radius.

`r=\frac{3.8\cdot(ft)/(\sec)}{0.314\cdot\frac{\text{rad}}{\sec }}=12.1ft`

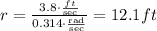
Therefore, the horse is 12.1 feet from the center.