Question

Graph the line y = - 2/5x +6

Answer 1

Given the equation of a line:

`y=-(2)/(5)x+6`

To graph the line, use the slop intercept form:

y = mx + b

Where m is the slope and b is the y-intercept.

Thus, the slope is:

`-(2)/(5)`

While the y-intercept is:

(0, 6)

Let's find the x-intercept.

Substitute y for 0 and solve for x to find the x-intercept.

We have:

`\begin{gathered} 0=-(2)/(5)x+6 \\ \\ \text{Multiply through by 5:} \\ 0(5)=-(2)/(5)x\ast5+6(5) \\ \\ 0=-2x+30 \\ \\ \text{Subtract 30 from both sides:} \\ 0-30=-2x+30-30 \\ \\ -30=-2x \\ \\ \text{divide both sides by -2:} \\ (-30)/(-2)=(-2x)/(-2) \\ \\ 15=x \end{gathered}`

Therefore, the x-intercept is; (15, 0)

Find the value of y when x is 5 and 10:

Substitute x for 5 and solve for y

`\begin{gathered} y=-(2)/(5)\ast5+6 \\ \\ y=-2+6 \\ \\ y=4 \\ \\ (5,4) \end{gathered}`
`\begin{gathered} y=-(2)/(5)\ast10+6 \\ \\ y=-4+6 \\ \\ y=2 \\ \\ (10,2) \end{gathered}`

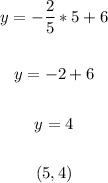
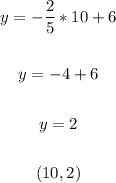
thus, we have the points:

(0, 6)

(5, 4)

(10, 2)

Mark the points on the graph and draw a straight line.

We have the graph below: