Question

Given the equation below graph the polynomial. On the graph indicate x and y intercepts multiplicity and end behavior.

Answer 1

Given the polynomial function h(x) defined as:

`h(x)=(x+3)^2(x-2)`

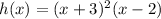
The y-intercept is the value of the function at x = 0. Then, evaluating h(0):

`\begin{gathered} h(0)=(0+3)^2(0-2)=3^2\cdot(-2)=-9\cdot2 \\ \Rightarrow h(0)=-18 \end{gathered}`

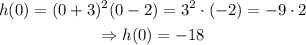
The y-intercept is a unique value, so its multiplicity is 1. On the other hand, the x-intercepts are those x-values such that h(x) = 0. Then, solving the polynomial equation for x:

`(x+3)^2(x-2)=0`

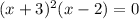
This equation is 0 for:

`\begin{gathered} (x+3)^2=0\Rightarrow x+3=0\Rightarrow x=-3 \\ (x-2)=0\Rightarrow x=2 \end{gathered}`

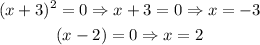
The first equation has a square exponent, so the multiplicity is 2. The multiplicity of the second equation is 1 because it is linear.

Summarizing:

x-intercepts:

i) -3, multiplicity 2

ii) 2, multiplicity 1

y-intercept:

i) -18, multiplicity 1

And the graph of the function looks like this:

For the end behavior, we need to analyze the limits for +∞ and -∞:

`\begin{gathered} \lim _(x\to+\infty)(x+3)^2(x-2)=\infty^2\cdot\infty=+\infty \\ \lim _(x\to-\infty)(x+3)^2(x-2)=(-\infty)^2\cdot(-\infty)=\infty\cdot(-\infty)=-\infty \end{gathered}`

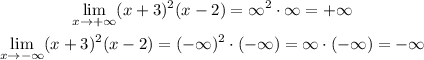
So the function tends to infinite when x tends to infinite, and to minus infinite when x tends to minus infinite.