Question

for the point (-4,4) on the terminal arm of the angle θ. a) find exact value of fractional form of sin θ

Answer 1

Given:

The point (-4,4) on the terminal arm of the angle θ.

Required:

Find exact value of fractional form of sin θ.

Step-by-step explanation:

Let x = -4

Let y = 4

Let r = the length of a line segment drawn from the origin to the point

`\begin{gathered} r=√(x^2+y^2) \\ r=√((-4)^2+4^2) \\ r=√(16+16) \\ r=√(32) \\ r=√(2*16) \\ r=4√(2) \end{gathered}`
`\begin{gathered} sin\theta=(y)/(r) \\ sin\theta=(4)/(4√(2)) \\ =(1)/(√(2)) \end{gathered}`

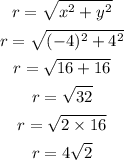
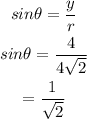
Answer:

This is the answer.