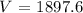Question:
Solution:
The volume of the cone with radius r and height h is given by the following formula:
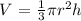
The data given in the problem are:
Diameter : 17.6 cm, then the radius is 17.6cm / 2 = 8.8 cm
Slant height : 25 cm
Thus, to find the height of the cone we use the Pythagorean theorem:
![h=\text{ }\sqrt[]{25^2-8.8^2}\text{ = 23.4}](https://img.qammunity.org/2023/formulas/mathematics/college/p9sxu4ytd30i5xd410tzuvr8f4b6n2zx8v.png)
then we have that the given cone has a height of 23.4 cm and a radius of 8.8cm. So that, replacing these that into the first equation (volume of the cone), we get:
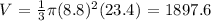
then, we can conclude that the correct answer is: