Question

A circle has a circumference of 8pi inches. Find its exact area.

Answer 1

Given that we know the circumference, first, we find the radius using the following formula

`\begin{gathered} C=2\pi r \\ 8\pi=2\pi r \end{gathered}`

Then, we solve for r

`\begin{gathered} r=(8\pi)/(2\pi) \\ r=4 \end{gathered}`

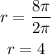
The radius of the circle is 4 inches long.

Now, we can find the area of the circle

`A=\pi(r)^2`

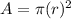
Where r = 4

`\begin{gathered} A=\pi(4in)^2 \\ A=16\pi(in)^2 \end{gathered}`

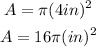
Therefore, the exact area of the circle is 16pi square inches.