Question

A1=-4; an= an-1+11What is the iterative rule for the arithmetic sequence above?

Answer 1

a1 = -4, means the first term of the sequaence = -4

In the arithmetic sequence, there is a common difference between each two consecutive terms

We can find this difference from the given rule

an = a n-1 + 11

an-1 is the previous term of an

11 is the common difference

The rule of the sequence is

`a_n=a+(n-1)d`

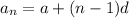
a is the first term

d is the common difference

n is the position of the term

Substitute a by -4

d by 11

`a_n=-4+(n-1)11`

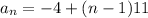
Multiply the bracket by 11

`\begin{gathered} a_n=-4+n(11)-1(11)_{} \\ a_n=-4+11n-11 \end{gathered}`

Add the like terms -4 and -11

`a_n=11n-15`

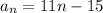
The rule of the sequence is a(n) = 11n - 15