Question

A formula for the normal systolic blood pressure for a man age A, measured in mmHg, is given as P = 0.006 A2 0.02 A + 120. Find the age of a man whose normal blood pressure measures 123 mmHg. Round your answer to the nearest year.

Answer 1

P = 0.006 A^2 - 0.02 A + 120

Where:

P = blood pressure

A = age

Replace P by 123 and solve for A

123 = 0.006 A^2 - 0.02 A + 120

0 = 0.006 A^2 - 0.02 A + 120 -123

0= 0.006 A^2 - 0.02 A - 3

The equation is in the form:

Ax^2+ b x + c

Where:

a = 0.006

b= -0.02

C= -3

Apply the quadratic formula:

`\frac{-b\pm\sqrt[]{b^2-4\cdot a\cdot c}}{2\cdot a}`

Replace:

`\frac{-(-0.02)\pm\sqrt[]{(-0.02)^2-4\cdot0.006\cdot-3}}{2\cdot0.006}`
`\frac{0.02\pm\sqrt[]{0.0004+0.072}}{0.012}`
`(0.02\pm0.26907248)/(0.012)`

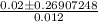
Positive:

(0.02+0.26907248) /0.012 = 24.1 years

Negative:

(0.02-0.026907248) /0.012 = -0.57

Since age can't be negative the answer is 24.1 years old