Answer:
26.6 degrees.
Explanation:
A triangle diagram representing the given problem is attached below:
In the smaller right triangle:
• The side ,opposite to ,angle x = 20 ft
,
• The side ,adjacent to ,angle x = 40 ft
From trigonometric ratios:
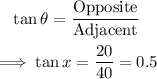
We then solve for x.
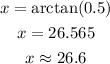
The value of x is 26.6 degrees (to the nearest tenth of a degree).