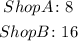Create a system of linear inequalities.
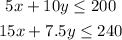
Multiply both sides of the first inequality by 3. Thus, we obtain the following:
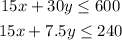
Subtract the second inequality from the first inequality.
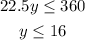
To obtain the value of x, substitute 16 in the first inequality.
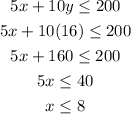
Solve for the number of hours per shop.
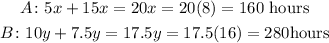
Thus,