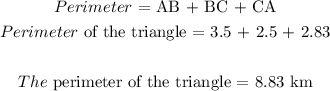Question

A triangle shaped Forrest is shaped by roads AB, BC, and CA per attached picture. Find the perimeter using the distance formula.

Answer 1

Answer:

The perimeter of the triangle is 8.83 km

Step-by-step explanation:

Given:

2) Triangle ABC with sides AB, BC and CA

To find:

The perimeter of the triangle using the distance formula

To determine the perimeter, we need to first find the 3 sides of the triangle. To do this, the distance formula will be used:

`$$dis\tan ce\text{ = }\sqrt[]{(y_2-y_1)^2+(x_2-x_1)^2}$$`

A = (0, 0), B = (3.5, 0) and C = (2, -2)

`\begin{gathered} Distance\text{ AB: A = \lparen0, 0\rparen, B = \lparen3.5, 0\rparen} \\ dis\tan ce\text{ AB = }√((0-0)^2+(3.5-0)^2)\text{ = }\sqrt{0\text{ + 12.25}} \\ distance\text{ AB = 3.5} \end{gathered}`
`\begin{gathered} Distance\text{ BC: B = \lparen3.5, 0\rparen and C = \lparen2, -2\rparen} \\ dis\tan ce\text{ = }√((-2-0)^2+(2-3.5)^2)\text{ = }√((-2)^2+(-1.5)^2) \\ distance\text{ = }\sqrt{4\text{ + 2.25}}\text{ = }√(6.25) \\ distance\text{ = 2.5} \end{gathered}`
`\begin{gathered} Distance\text{ CA = C = \lparen2, -2\rparen and A = \lparen0, 0\rparen} \\ dis\tan ce\text{ CA = }√((0-(-2))^2+(0-2)^2)\text{ = }√((0+2)^2+(-2)^2) \\ distance\text{ CA}=\text{ }√(4+4)\text{ = }√(8) \\ distance\text{ CA = 2.83} \end{gathered}`

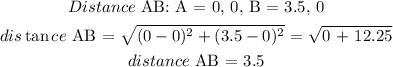
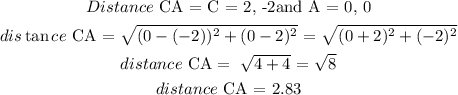
The perimeter of the triangle = sum of all 3 sides

`\begin{gathered} Perimeter\text{ = AB + BC + CA} \\ Perimeter\text{ of the triangle = 3.5 + 2.5 + 2.83} \\ \\ The\text{ perimeter of the triangle = 8.83 km} \end{gathered}`