Question

I need help with this problem please.Find the vertex and equation of the axis of symmetry.

Answer 1

First, let's expand the given equation:

`\text{ f(x) = }-(x-7)^2\text{ - }29`
`\text{ f(x) = }-(x^2-14x+49)^{}\text{ - }29`
`\text{ f(x) = }-x^2+14x-49\text{ - }29`
`\text{ f(x) = }-x^2+14x-78`

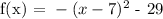
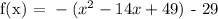
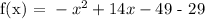
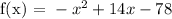
We get, a = -1, b = 14 and c= -78

A.) Let's determine the vertex.

`\text{ x = }(-b)/(2a)\text{ = }(-(14))/(2(-1))\text{ = }(-14)/(-2)`
`\text{ x = 7}`

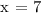
Substituting x = 7 in the equation, let's find y.

`y\text{ = }-(x-7)^2\text{ - }29`
`y\text{ = }-(7-7)^2\text{ - }29`
`y\text{ = }-(0)^2\text{ - }29`
`\text{ y = -29}`

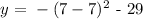
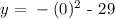
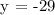
Therefore, the coordinate of the vertex is x,y = 7, -29

B. Let's determine the equation of the axis of symmetry.

The equation for the axis of symmetry of a parabola can be expressed as:

`\text{ x = }(-b)/(2a)`

We get,

`\text{ x = }(-b)/(2a)\text{ = }(-(14))/(2(-1))\text{ = }(-14)/(-2)`
`x\text{ = 7}`

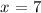
Therefore, the equation for the axis of symmetry is x = 7.