Question

On a given planet the weight of an object varies directly with the mass of the object. Suppose that an object whose mass is 80 KG weighs 80 n calculate the mass of another object that weighs 70 n

Answer 1

Step-by-step explanation

We are told that the weight of an object varies directly with the mass of the object. This basically means that if w is the weight and M is the mass then these two magnitudes are related by the following expression:

`w=k\cdot M`

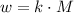
Where k is a constant.

We know that an object with a mass of 80kg weights 80 newtons. Then for this object we have w=80kg and M=80n so we get:

`80n=k\cdot80kg`

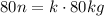
We divide both sides of this equation by 80:

`\begin{gathered} (80n)/(80)=(k\cdot80kg)/(80) \\ 1n=k\cdot1kg \end{gathered}`

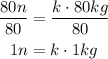
Here it's important to remember that 1 newton (i.e. 1n) is equal to:

`n=(kg\cdot m)/(s^2)`

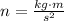
Then we get:

`1(kg\cdot m)/(s^2)=k\cdot1kg`

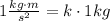
We divide both sides by 1 kg:

`\begin{gathered} (1(kg\cdot m)/(s^2))/(1kg)=(k\cdot1kg)/(1kg) \\ 1(kg\cdot m)/(kg\cdot s^2)=k\cdot(1kg)/(1kg) \\ k=1(m)/(s^2) \end{gathered}`

So now that we found k we have the following expression for the weight of an object as a function of its mass:

`w=M\cdot1(m)/(s^2)`

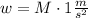
For an object that weights 70n we get:

`\begin{gathered} 70n=M\cdot1(m)/(s^2) \\ 70(kg\cdot m)/(s^2)=M\cdot(m)/(s^2) \end{gathered}`

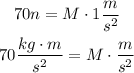
We divide both sides by 1 m/s²:

`\begin{gathered} (70(kg\cdot m)/(s^2))/(1(m)/(s^2))=(M\cdot(m)/(s^2))/(1(m)/(s^2)) \\ 70(kg\cdot m\cdot s^2)/(m\cdot s^2)=M\cdot(m\cdot s^2)/(s^2\cdot m) \\ 70kg=M \end{gathered}`

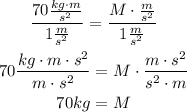
Answer

So the mass of an object that weights 70n is 70kg.