Question

find the domain of the following equationsfor the domain I got
`x \ \textgreater \ 0`and x cannot be equal to one but it said my answers were wrong

Answer 1

To find the domain of a function, look for the restrictions over the functions involved in it.

`\text{Let }f(x)=\log _3(\sqrt[]{x}+1)`

The log functions are defined whenever their argument is greater than 0. This means:

`\sqrt[]{x}+1>0`

Since 1>0 and the square root of x is always greater than or equal to 0, then this does not restrict the domain of the function.

Nevertheless, the square root of x requires that:

`x\ge0`

This is the only restriction over the variable x.

Therefore, the domain of the function, is:

`0\leq x`

Next, solve the equation:

`\log _3(\sqrt[]{x}+1)=1`

Notice that given this equation, then 3 to the power of each side of the equation should be equal:

`3^{\log _3(\sqrt[]{x}+1)}=3^1`

Use the fact that:

`3^(\log _3(a))=a`

to simplify the equation:

`\begin{gathered} 3^{\log _3(\sqrt[]{x}+1)}=3^1 \\ \Rightarrow \\ \sqrt[]{x}+1=3^1 \end{gathered}`

Simplify the power 3^1:

`\sqrt[]{x}+1=3`

Substract 1 from both sides of the equation:

`\sqrt[]{x}=2`

Square both sides of the equation:

`(\sqrt[]{x})^2=2^2`

Simplify the powers:

`x=4`

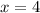
Check the answer by plugging in x=4 into the equation:

`\begin{gathered} \log _3(\sqrt[]{x}+1)=1 \\ \Rightarrow \\ \log _3(\sqrt[]{4}+1)=1 \\ \Rightarrow \\ \log _3(2+1)=1 \\ \Rightarrow \\ \log _3(3)=1 \\ \Rightarrow \\ 1=1 \end{gathered}`

Since we got an identity, the answer x=4 is correct.