Explanation
Part A
Part B
The relationship between two variables is generally considered strong when their r value is larger than 0.7. The correlation r measures the strength of the linear relationship between two quantitative variables.
Therefore the relationship is not very strong with the calculated model.
Part C
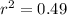
The coefficient of determination is a measure used in statistical analysis to assess how well a model explains and predicts future outcomes. while a value of 0.49 suggests that 49% of the dependent variable is predicted by the independent variable, and so forth.
Part D
The slope of the regression is 0.29 which means that for each new adult the percentage return increases by 0.29.
Part E
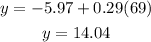
The new adult is 14