Question

Identify all values of x that make the equation true.a. x=25/xb. x+2=6x-3/xc. x/x^2=3/xd. 6x^2+18x/2x^3=5/x

Answer 1

The values of x that make the equation true are;

1. x = ±5

2. x = 3 or x = 1

3.
`x = ±\sqrt{ \frac{ {x}^(2) }{3} }`

4. x = 0 or 4.5

Which values of x that make the equation true?

1.

`x = (25)/(x)`

cross product

x² = 25

find the square root of both sides

x = √25

x = ±5

2.

`x + 2 = (6x - 3)/(x)`

cross product

x(x + 2) = 6x - 3

x² + 2x = 6x - 3

x² + 2x - 6x + 3 = 0

x² - 4x + 3 = 0

x² -3x - x + 3 = 0

x(x -3) -1(x - 3) = 0

(x -3) (x - 1) = 0

x - 3 = 0 or x - 1 = 0

x = 3 or x = 1

3.

`\frac{x}{ {x}^(2) } = (3)/(x)`

cross product

x × x = 3 × x²

x² = 3x²

Divide both sides by 3

`\frac{ {x}^(2) }{3} = {x}^(2)`

Find the square root of both sides

`x = ±\sqrt{ \frac{ {x}^(2) }{3} }`

4.

`\frac{ {6x}^(2) + 18x }{ {2x}^(3) } = (5)/(x)`

cross product

x(6x² + 18x) = 5(2x³)

6x³ + 18x² = 10x³

6x³ + 18x² - 10x³ = 0

-4x³ + 18x² = 0

-2x²(2x - 9) = 0

-2x² = 0 or 2x - 9 = 0

x = 0 or 2x = 9

x = 0 or x = 9/2

x = 0 or 4.5

Answer 2

We want to solve the following equations:

item (a):

`x=(25)/(x)`

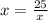
To solve this one, let's start by multiplying both sides by x.

`x^2=25`

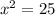
Now, let's take the square root of both sides

`\begin{gathered} \sqrt[]{x^2}=\sqrt[]{25} \\ x=\pm5 \end{gathered}`

The solutions for this equation are x = 5 and x = -5.

item (b):

`x+2=(6x-3)/(x)`

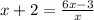
To solve this one, let's again start by multiplying both sides by x.

`\begin{gathered} x(x+2)=6x-3 \\ x^2+2x=6x-3 \end{gathered}`

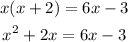
Now, let's subtract 2x from both sides.

`\begin{gathered} x^2+2x-2x=6x-3-2x \\ x^2=4x-3 \end{gathered}`

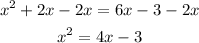
Let's rewrite this equation with all terms in the right side

`x^2-4x+3=0`

Factorizing

`\begin{gathered} x^2-4x+3=(x-1)(x-3) \\ \Rightarrow(x-1)(x-3)=0 \end{gathered}`

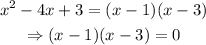
Since this is a product of two terms, the result will be zero only if one of them is zero.

Then, we get two equations

`\begin{gathered} x-1=0 \\ x-3=0 \end{gathered}`

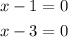
The solutions for those two equations are the solutions for our system.

`\begin{gathered} x-1=0\Rightarrow x=1 \\ x-3=0\Rightarrow x=3 \end{gathered}`

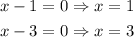
The solutions for this equation are x = 1 and x = 3.

item (c):

`(x)/(x^2)=(3)/(x)`

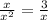
Let's start by solving the division in the right side

`\begin{gathered} (x)/(x^2)=x^(1-2)=x^{-1^{}}=(1)/(x) \\ \Rightarrow(1)/(x)=(3)/(x) \end{gathered}`

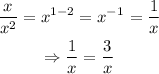
Multiplying both sides by x, we have:

`1=3`

Since this statement is false, this equation have no solution.

item (d):

`(6x^2+18x)/(2x^3)=(5)/(x)`

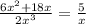
Let's start by multiplying both sides by x.

`(6x^2+18x)/(2x^2)=5`

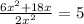
Doing the division on the left side of the equality, we have:

`\begin{gathered} (6x^2+18x)/(2x^2)=(6x^2)/(2x^2)+(18x)/(2x^2)=3+(9)/(x) \\ \Rightarrow3+(9)/(x)=5 \end{gathered}`

Subtracting 3 from both sides:

`(9)/(x)=2`

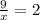
Multiplying both sides by x again:

`\begin{gathered} 9=2x \\ x=(9)/(2)=4.5 \end{gathered}`

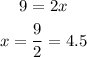
The solution for this system is x = 4.5.