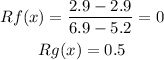Answer:

Explanation:
To determine the average rate of change of an interval [a,b], we must use the following equation:
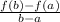
Therefore, for the interval [0,4]:
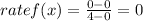
For g(x) we need to find the equation of the line by the slope-point form:
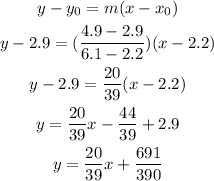
To find the value to x=4, substitute it into the equation:

Now, for [0,8]:
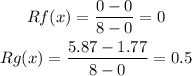
[0,2.2]:
Since g(x) is a linear function, it will have a constant rate of change 0.5.
f(x):

[5.2, 6.1]:
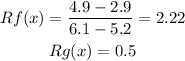
[5.2,6.9]: