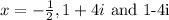Question

Using the tools you learned in this lesson, find all solutions (real and non-real) for the polynomial: 2x^3-3x^2+32x+17 To earn full credit please share all work, calculations and thinking. If you prefer you can do the work by hand on a piece of paper, take a picture of that work and upload it.

Answer 1

The given polynomial function is;

`f(x)=2x^3-3x^2+32x+17`

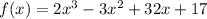
Firstly, we would find a factor of the function by a trial and error method.

Thus, we have:

`\begin{gathered} \text{when x=-1} \\ f(-1)=2(-1)^3-3(-1)^2+32(-1)+17 \\ f(-1)=2(-1)-3(1)-32+17 \\ f(-1)=-2-3-32+17 \\ f(-1)=-20 \\ \text{Thus,(x}+1)\text{ is not factor} \end{gathered}`
`\begin{gathered} \text{when x=}(-1)/(2) \\ f(-(1)/(2))=2(-(1)/(2))^3-3(-(1)/(2))^2+32(-(1)/(2))+17 \\ f(-(1)/(2))=2(-(1)/(8))-3((1)/(4))-(32)/(2)+17 \\ f(-(1)/(2))=-(2)/(8)-(3)/(4)-(32)/(2)+(17)/(1) \\ f(-(1)/(2))=(-2-6-128+136)/(8) \\ f(-(1)/(2))=(0)/(8)=0 \\ \text{Since, this is equal to zero, it implies that;} \\ 2x+1\text{ is a factor of the given polynomial} \end{gathered}`

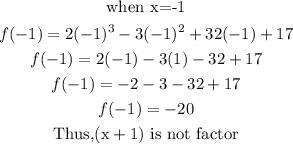
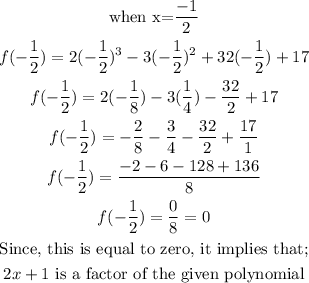
We are going to use the long division method to get the other factor(s).

Thus, we have:

The final remainder of the long division is 0.

Thus, the factors of the polynomial are:

`(2x+1)and(x^2-2x+17)`

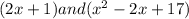
Find the zeros of these factors, we have:

`\begin{gathered} 2x+1=0 \\ 2x=-1 \\ x=-(1)/(2) \end{gathered}`
`\begin{gathered} x^2-2x+17 \\ \text{This is not factorizable, thus we would make use of the quadratic formula to solve it} \end{gathered}`

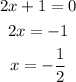
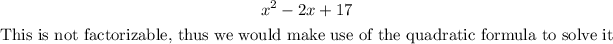
The quadratic formula is given by the equation;

`x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}`
`\begin{gathered} \text{From the equation;} \\ x^2-2x+17 \\ a=1;b=-2;c=17 \\ \text{Thus, we have} \\ x=\frac{-(-2)\pm\sqrt[]{(-2)^2-4(1)(17)}}{2(1)} \\ x=\frac{2\pm\sqrt[]{4-68}}{2} \\ x=\frac{2\pm\sqrt[]{-64}}{2} \\ \text{Recall from complex number;} \\ i=\sqrt[]{-1} \\ \text{Thus, }\sqrt[]{-64}\text{ can be further expressed as }\sqrt[]{64}\text{ }*\sqrt[]{-1} \\ \Rightarrow8i \\ \text{Thus, } \\ x=(2\pm8i)/(2) \\ x=(2+8i)/(2)\text{ OR }(2-8i)/(2) \\ x=(2(1+4i))/(2)\text{ OR }(2(1-4i))/(2) \\ x=1+4i_{} \\ OR \\ x=1-4i \end{gathered}`

Hence, the solutions are:

`x=-(1)/(2),1+4i\text{ and 1-4i}`