Given:
Tobias has 2 times as many quarters as nickels.
They have a combined value of 275 cents.
To find:
The number of each coin.
Step-by-step explanation:
Let q be the number of quarters.
Let n be the number of nickels.
Since he has 2 times as many quarters as nickels.
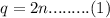
We know that,
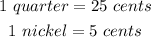
According to the problem,
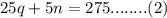
Substituting equation (1) in (2), we get
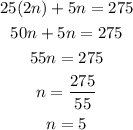
From (1), we get
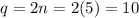
Therefore, the number of quarters is 10 and the number of nickels is 5.
Final answer:
• The number of quarters is 10.
,
• The number of nickels is 5.