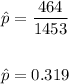Question

Suppose a sample of 1453 tankers is drawn. Of these ships 989 did not have spills. Using the data estimate the proportion of all tankers that had spills. Answer your answer as a fraction or a decimal number rounded to three decimal places.

Answer 1

We have the following situation:

• We have a sample of 1453, n = 1453.

• Of these ships, 989 did not have spills.

And from this, we need to estimate the proportion of oil tankers that had spills.

To find it, we can proceed as follows:

1. We need to find the sample proportion of ships that had spills. These ships are:

`\begin{gathered} 989\text{ ships did not have spills from 1453.} \\ \text{ Then the ships that have spills are:} \\ 1453-989=464 \end{gathered}`

2. Then the sample proportion is given by:

`\begin{gathered} \hat{p}=(464)/(1453)=0.319339298004 \\ \\ \text{ If we round this value to a decimal number rounded to three decimal places: } \\ \\ \hat{p}=0.319 \end{gathered}`

Therefore, in summary, the proportion of oil tankers that had spills is (as a fraction or decimal with three places):

`\begin{gathered} \hat{p}=(464)/(1453) \\ \\ \hat{p}=0.319 \end{gathered}`