Question

Q3. Find the height of the top of the tower (labelled x) given the following scenario:I’m confused on how I should do it

Answer 1

The Solution:

Given the diagram below:

Considering right-angled triangle ABC,

we can apply the Trigonometrical Ratio as below:

`\begin{gathered} \tan 25^o=(y)/(87) \\ \text{Cross multiplying, we get} \\ y=87\tan 25^o\ldots eqn(1) \end{gathered}`

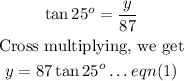
Similarly, considering right-angled triangle ABD,

we can apply the Trigonometrical Ratio as below:

`\begin{gathered} \tan 40^o=(x+y)/(87) \\ \text{Cross multiplying, we get} \\ x+y=87\tan 40^o\ldots eqn(2) \end{gathered}`

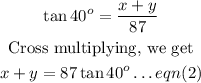
Now, putting eqn(1) into eqn(2), we get

`x+87\tan 25^o=87\tan 40^o`

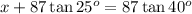
Solving for x, we have

`\begin{gathered} x=87\tan 40^o-87\tan 25^o \\ x=87(\tan 40^o-\tan 25^o) \end{gathered}`
`x=87(0.8390996-0.4663077)=87*0.37279=32.4329\approx32.43\text{ feet}`

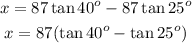
Therefore, the correct answer is 32.43 feet.