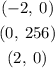Step 1
Given;

Required; To find the coordinates of the local minima and maxima
Step 2
Find the local minima and maxima

Therefore; f'(x) is given as;
![f^(\prime)(x)=2x(x^2-4)^3(x^2+1)^4[9x^2-16]](https://img.qammunity.org/2023/formulas/mathematics/college/hom3cj8qs8euu04ah7wcjy4kz0gdeakxsq.png)
Step 3
Find the increasing and decreasing intervals from the graph
![\begin{gathered} Decreasing;-\inftyPlugin x=-2 into y[tex]\begin{gathered} \mathrm{Minimum}\left(-2,\:0\right) \\ \end{gathered}]()
Plugin -4/3 into y
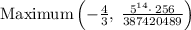
Plugin x=0 into y
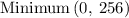
Plugin x=4/3
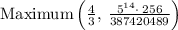
Plugging x=2 into y
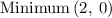
Answer; The maximum points are;
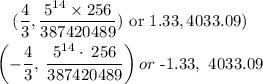
The minimum points are ;