Question

Question 10 with a thorough explanation please

Answer 1

1) Find the perimeters of the square and the triangle.

Suppose you divide the wire into 2 pieces that measure x and y. x is used to make the square and y is used to make the triangle, according to the figure:

x is the perimeter of the square;

y is the perimeter of the triangle.

Since the total wire is 30 inches long:

x + y = 30 (equation 1).

2) Find the area of the geometric figures.

Square:

Since a square has 4 equal sides, each side will measure x/4 (4*x/4=x)

And, since the area (As) of a square whose side measures a is:

`As=a^2`

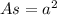
The area of the square is:

`As=((x)/(4))^2=(x^2)/(16)`

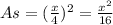
Equilateral Triangle:

Since an equilateral triangle has 3 equal sides, each side will measure y/3 (3*y/3 = y).

And, since the area (At) of an equilateral triangle whose side measures b is:

`At=\frac{\sqrt[]{3}}{4}\cdot b^2`

The area of the triangle is:

`\begin{gathered} At=\frac{\sqrt[]{3}}{4}\cdot((y)/(3))^2 \\ At=\frac{\sqrt[]{3}}{4}\cdot(y^2)/(9)=\frac{\sqrt[]{3}}{36}\cdot y^2 \end{gathered}`

3) Find the total area.

The total area (A) is the sum of the areas:

`\begin{gathered} A=As+At \\ A=(x^2)/(16)+\frac{\sqrt[]{3}}{36}\cdot y^2 \end{gathered}`

4) Isolate y in equation 1 and substitute in the equation for the area.

x + y = 30

y = 30 - x

`A=(x^2)/(16)+\frac{\sqrt[]{3}}{36}\cdot(30-x)^2`

5) The maximum or minimum value of A happens at x when dA/dx = 0.

So, derivate A:

`\begin{gathered} (dA)/(dx)=(2x)/(16)+\frac{\sqrt[]{3}}{36}\cdot(30-x)\cdot2\cdot(-1) \\ (dA)/(dx)=(x)/(8)-\frac{\sqrt[]{3}}{18}(30-x) \end{gathered}`

And do dA/dx = 0 to find x.

`0=(x)/(8)-\frac{\sqrt[]{3}}{18}(30-x)`

Then, isolate x:

`\begin{gathered} 0=(x)/(8)-\frac{\sqrt[]{3}}{18}\cdot30+\frac{\sqrt[]{3}}{18}\cdot x \\ \frac{\sqrt[]{3}}{18}\cdot30=(x)/(8)+\frac{\sqrt[]{3}}{18}\cdot x \\ 2.89=0.3x \\ x=(2.89)/(0.22) \\ x=13 \end{gathered}`

6) Evaluate the equation for x at the exremes x = 0; y = 30, and at x = 13.

For x = 0

`\begin{gathered} A=(x^2)/(16)+\frac{\sqrt[]{3}}{36}\cdot(30-x)^2 \\ (0^2)/(16)+\frac{\sqrt[]{3}}{36}\cdot(30-0)^2 \\ =\frac{\sqrt[]{3}}{36}\cdot900 \\ =43.3 \end{gathered}`

For x = 30

`\begin{gathered} A=(x^2)/(16)+\frac{\sqrt[]{3}}{36}\cdot(30-x)^2 \\ A=(30^2)/(16)+\frac{\sqrt[]{3}}{36}\cdot(30-30)^2 \\ =(900)/(16)+\frac{\sqrt[]{3}}{36}\cdot0 \\ =56.25 \end{gathered}`

And for x =13

`\begin{gathered} A=(13^2)/(16)+\frac{\sqrt[]{3}}{36}\cdot(30-13)^2 \\ A=(169)/(16)+\frac{\sqrt[]{3}}{36}\cdot17^2 \\ A=(169)/(16)+\frac{\sqrt[]{3}}{36}\cdot289 \\ A=24.47 \end{gathered}`

7) Compare the results:

As you can see in step 6, when x = 13, the area is minimized, while the area is maximized when x = 30.

The greatest area will be reached when x = 30. That means y = 0 (x + y =30).

Answer:

The greatest area is reached when all the wire is used to make the square.

To make the 2 figures, we wire should be cut as nearest as possible to 30, and the greatest piece should be used to make the square.