Question

Select all of the steps that are possible when solving for X

Answer 1

We need to solve the following expression:

`5(1)/(2)x+(2)/(3)x=37`

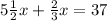
To find which steps are possible we will solve the equation one step at a time and check which of these steps appear on the options.

The first step is to transform the mixed fraction into a improper fraction, we do that by adding the integer part wih the fraction part.

`\begin{gathered} (5+(1)/(2))x+(2)/(3)x=37 \\ ((2\cdot5+1)/(2))x+(2)/(3)x=37 \\ ((10+1)/(2))x+(2)/(3)x=37 \\ (11)/(2)x+(2)/(3)x=37 \end{gathered}`

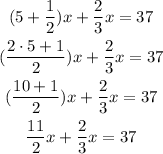
The second step is to find the LCM of the two fractions and add them.

`\begin{gathered} (11\cdot3\cdot x+2\cdot2\cdot x)/(6)=37 \\ (33x+4x)/(6)=37 \\ (37x)/(6)=37 \end{gathered}`

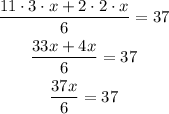
The third step is to multiply both sides by 6.

`\begin{gathered} (37x)/(6)\cdot6=37\cdot6 \\ 37x=222 \end{gathered}`

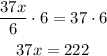
The fourth step is to divide both sides by 37.

`\begin{gathered} (37)/(37)x=(222)/(37) \\ x=6 \end{gathered}`

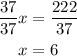
The first, second and fourth step appear on the options. Therefore we should marke the option "x=6", the option "37/6 x=37" and the option "11/2 x + 2/3 x= 37".