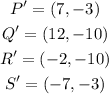Get the coordinates of PQRS, observing the graph, the coordinates are: P = (-3,7), Q = (4,12), R = (4,-2) and S = (-3,-7)
First apply the translation by 6 units to the right. We apply this by adding 6 units to the x values of the points
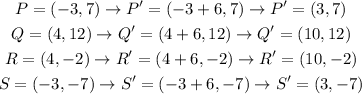
Next apply the rotation transformation which transforms (x , y) into (y , -x)
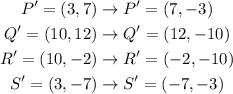
Therefore, the final points for P'Q'R'S' is