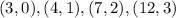Question

Consider the following functions f(x) = sqrt(x - 3) and g(x) = 4 Step 1 of 3Find four ordered pairs that satisfy the function, f(x) = sqrt(x - 3)

Answer 1

Given:

`f(x)=√(x-3)`

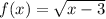
To find:

Find the four ordered pairs that satisfy the given equation.

Step-by-step explanation:

The domain of a function is the set of input or argument values for which the function is real and defined.

For the given expression, let find the non-negative value for radicals,

x - 3 is in square root form, so,

`x\ge3`

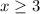
Now, taking values of x that is equal to 3 or more than 3:

For x = 3, the value of y is:

`f(3)=√(3-3)=0`

For x = 4, the value of y is:

`f(4)=√(4-3)=1`

For x = 7, the value of y is:

`f(7)=√(7-3)=√(4)=2`

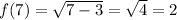
For x = 12, the value of y is:

`f(12)=√(12-3)=√(9)=3`

So, we can summarize the coordinate pairs in table form as shown below:

Final answer:

Hence, the required four coordinate pairs that satisfy the given function is:

`(3,0),(4,1),(7,2),(12,3)`