Question

Determine the concavity of the graph of f(x) = 8 - x^2 between x = -1 and x = 5 by calculating average rates of change over intervals of length 2.a) The average rate of change over the interval -1 ≤ x ≤ 1 =b) The average rate of change over the interval 1 ≤ x ≤ 3=c) The average rate of change over the interval 3 ≤ 2 ≤ 5 =

Answer 1

Given the function below

`f(x)=8-x^2`

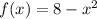
To determine the concavity of the graph of the given function

We calculate the average rate change of the given interval

The formula to find the average rate of change, A(x), change is

`A(x)=(f(b)-f(a))/(b-a)`

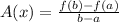
a) For the interval -1 ≤ x ≤ 1, the average rate of change, A(x) is

`\begin{gathered} A(x)=(f(b)-f(a))/(b-a) \\ \text{Where a}=-1,b=1,f(x)=8-x^2 \\ A(x)=((8-(1)^2)-(8-(-1)^2))/(1-(-1))=((8-1)-(8-1))/(1+1)=(7-7)/(2)=(0)/(2)=0 \end{gathered}`

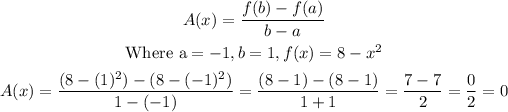
Hence, the average rate change over the interval -1 ≤ x ≤ 1 is 0

b) For the interval 1 ≤ x ≤ 3, the average rate of change, A(x) is

`\begin{gathered} A(x)=(f(b)-f(a))/(b-a) \\ \text{Where a}=1,b=3,f(x)=8-x^2 \\ A(x)=((8-(3)^2)-(8-(1)^2))/(3-1) \\ A(x)=((8-9)-(8-1))/(2) \\ A(x)=(-1-(7))/(2) \\ A(x)=(-8)/(2) \\ A(x)=-4 \end{gathered}`

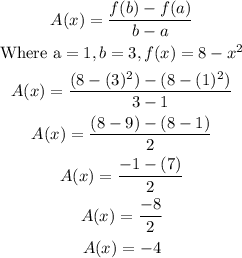
Hence, the average rate change over the interval 1 ≤ x ≤ 3 is -4

c) For the interval 3 ≤ x ≤ 5, the average rate of change, A(x) is

`\begin{gathered} A(x)=(f(b)-f(a))/(b-a) \\ \text{Where a}=3,b=5,f(x)=8-x^2 \\ A(x)=((8-(5)^2)-(8-(3)^2))/(5-3) \\ A(x)=((8-25)-(8-9))/(2) \\ A(x)=(-17-(-1))/(2) \\ A(x)=(-17+1)/(2) \\ A(x)=(-16)/(2) \\ A(x)=-8 \end{gathered}`

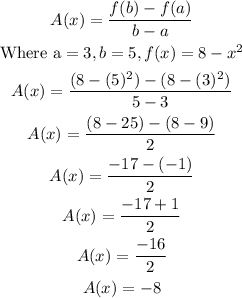
Hence, the average rate change over the interval 3 ≤ x ≤ 5 is -8

d) From the above deductions, the average rate of change is decreasing.

Hence, the graph of the function f(x) is Concave down