Question

D 2 in F 12 in E 2 in, This trapezoid has been divided into two right triangles and a rectangle 9 in. How can the area of the trapezoid be determined using the area of each shape? Enter your answers in the boxes, A 12 in. The area of the triangle on left is 108 in?, the area of the triangle on the right is 18 in", and the area of the rectangle 15 126 in? The area of the trapezoid is the sum of these areas, which is 192 in .

Answer 1

The area of the triangle on the left will be calculated using the image below

Concept:

The area of the triangle is given below

`\begin{gathered} A_{\text{traingle}}=(1)/(2)* base* height \\ \text{base}=2in \\ \text{height}=9in \end{gathered}`

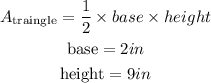
By substituting the values, we will have

`\begin{gathered} A_{\text{traingle}}=(1)/(2)* base* height \\ A_{\text{traingle}}=(1)/(2)*2in*9in \\ A_{\text{traingle}}=(18in^2)/(2) \\ A_{\text{traingle}}=9in^2 \end{gathered}`

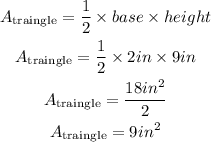
Hence,

The area of the triangle on the left = 9in²

Step 2:

The image below will be used to calculate the area of triangle on the right

The area of the triangle is given below

`\begin{gathered} A_{\text{traingle}}=(1)/(2)* base* height \\ \text{base}=2in \\ \text{height}=9in \end{gathered}`

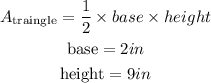
By substituting the values, we will have

`\begin{gathered} A_{\text{traingle}}=(1)/(2)* base* height \\ A_{\text{traingle}}=(1)/(2)*2in*9in \\ A_{\text{traingle}}=(18in^2)/(2) \\ A_{\text{traingle}}=9in^2 \end{gathered}`

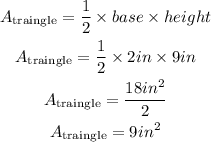
Hence,

The area of the triangle on the right = 9in²

Step 3:

The image below represents the rectangle

The area of the rectangle is calculated below as

`\begin{gathered} A_{\text{rectangle}}=\text{length}* breadth \\ \text{where,} \\ \text{length}=12in \\ \text{breadth}=9in \end{gathered}`

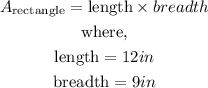
By substituting the values, we will have

`\begin{gathered} A_{\text{rectangle}}=\text{length}* breadth \\ A_{\text{rectangle}}=12in*9in \\ A_{\text{rectangle}}=108in^2 \end{gathered}`

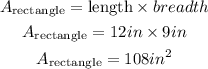
Hence,

The area of the rectangle is =108 in²

The area of the trapezoid will be calculated using the formula below

`\begin{gathered} A_{\text{trapezoid}}=A_{\text{traingle}}+A_{\text{triangle}}+A_{\text{recatngle}} \\ A_{\text{trapezoid}}=9in^2+9in^2+108in^2 \\ A_{\text{trapezoid}}=126in^2 \end{gathered}`

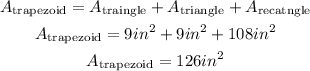
Hence,

The area of the trapezoid is = 126 in²