Given:
• Number of identical charges = 3
,
• Charge, q1 = q2 = q3 = 6.39 x 10⁻⁴ C.
,
• Length of each side of the triangle, d = 3.35 m
,
• θ = 60 degrees
Let's find the magnitude of the net force on q3.
For forces acting on q3 due to q1 and q2, apply the formula:
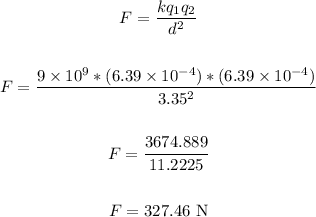
The magnitude of net force on all 3 charges will also be equal.
Now, to find the magnitude of the net force acting on q3, apply the formula:

Thus, we have:

Therefore, the magnitude of net force acting on charge 3 is 567.18 N.
• ANSWER:
567.18 N