Pair A:
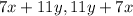
Remember that characteristic of the numbers is that, in general:
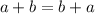
For any numbers a and b, this is always true. So, in our case:
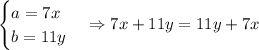
So the pair A is equivalent
As for pair B:
We are going to it by contradiction: if we suppose that the two equations are the same (one is equal to the other) and we found a pair of numbers (x,y) that produce a contradiction, then the two equations cannot be equivalent. Let me show you:
Suppose pair B is equivalent, then:
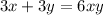
Now, suppose that x=0 and y=1, then:
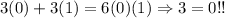
And of course, 3 is not equal to 0!
So, by supposing that the 2 equations are equivalent we reach a false implication, which means that the pair is NOT equivalent
As for pair C:
We can expand the expression 6(2x-y):
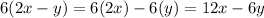
Which is exactly the first expression! So the pair is equivalent!