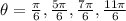Question

Hello, how do I solve these equations when the domain is restricted to 0 ≤ θ <2πa) 5cot(θ) -2 = 3cot(θ) - 2b) 2sinθ = tanθc) 3cos^2θ - sin^2θ = 2

Answer 1

a)

The given equation is

`5cot\theta-2=3cot\theta-2`

Subtract 3cot(theta) from each side

`\begin{gathered} 5cot\theta-3cot\theta-2=3cot\theta-3cot\theta-2 \\ 2cot\theta-2=-2 \end{gathered}`

Add 2 to both sides

`\begin{gathered} 2cot\theta-2+2=-2+2 \\ 2cot\theta=0 \end{gathered}`

Divide both sides by 2

`\begin{gathered} (2cot\theta)/(2)=(0)/(2) \\ \\ cot\theta=0 \end{gathered}`

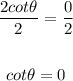
cot(theta) = 0 at theta = pi and theta = 3/2pi

`\theta=\pi,(3)/(2)\pi`

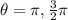
b)

`2sin\theta=tan\theta`

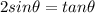
Change tan(theta) to sin(theta)/cos(theta)

`\begin{gathered} tan\theta=(sin\theta)/(cos\theta) \\ \\ 2sin\theta=(sin\theta)/(cos\theta) \end{gathered}`

Multiply both sides by cos(theta)

`2sin\theta cos\theta=sin\theta`

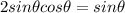
Subtract sin(theta) from both sides

`2sin\theta cos\theta-sin\theta=0`

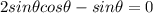
Take sin(theta) as a common factor on the left side

`sin\theta(2cos\theta-1)=0`

Equate each factor by 0

`\begin{gathered} sin\theta=0 \\ \theta=0,\pi \end{gathered}`

`\begin{gathered} 2cos\theta-1=0 \\ 2cos\theta=1 \\ cos\theta=(1)/(2) \\ \theta=(\pi)/(3),(5\pi)/(3) \end{gathered}`
`\theta=0,(\pi)/(3),\pi,(5\pi)/(3)`

c)

`3cos^2(\theta)-sin^2(\theta)=2`

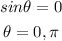
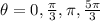
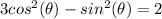
Change sin^2(theta) to 1 - cos^2(theta)

`\begin{gathered} 3cos^2\theta-(1-cos^2\theta)=2 \\ 3cos^2\theta-1+cos^2\theta=2 \\ 4cos^2\theta-1=2 \\ 4cos^2\theta=3 \\ cos^2\theta=(3)/(4) \end{gathered}`

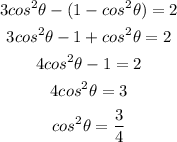
Take a square root for each side

`cos\theta=-(√(3))/(2),cos\theta=(√(3))/(2)`

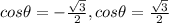
The values of theta are

`\theta=(\pi)/(6),(5\pi)/(6),(7\pi)/(6),(11\pi)/(6)`