From the property of lines :
If two lines are perpendicular then, the product of the slope of both the lines are equal to ( - 1)
where slope of line is express as :
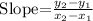
For the slope of the given line,
consider any two coordinates : (-2, 0) & (0,4)
Substitute the coordinates in the expression of the slope,
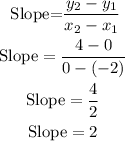
Slope = 2
Let the slope of the given line is express as m i.e. m = 2
Consider the slope of the line which is perpendicular to given line f is n
Apply the property of line :
Product of slope = (- 1 )
m x n = ( - 1)
Substitute the values :
2 x n = ( -1)
n = (- 1) /2
Slope of the line which is perpendicular to the line f is (-1/2)
Answer : Slope of the line which is perpendicular to the line f is (-1/2)