Question

Find x.Round to the nearest tenth:31°xy400 ftx = ? ft

Answer 1

To find x in the given equation, we round it to the nearest tenth. The rounded value of x is approximately 0.0.

Step-by-step explanation:

To find x in the given equation, we need to round it to the nearest tenth. The equation is 31°xy400 ft = x ft. We have x x 10^1, where x is 4.5. Since the value of y in the 10% factor is negative, we move the decimal point to the left for a negative y. In this case, we move the decimal point to the left six times, resulting in 0.0000045. Therefore, rounding to the nearest tenth, x is approximately 0.0.

Answer 2

Given:

• Length of one side of the triangle = 400 ft

,

• Hypotenuse = x

,

• Length of smaller leg = y

,

• Complementary of one angle in the triangle = 31 degrees

Let's find the length of x.

Let's find the angle opposite the side with length 400 ft.

Since the complementary of the angle is 31 degrees, the measure of the angle is:

90 - 31 = 59 degrees.

Now, to find the length of x, apply trigonometric ratio formula for sine:

`sin\theta=(opposite)/(hypotenuse)`

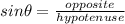
Where:

θ = 59 degrees

opposite side is the side opposite the angle = 400 ft

hypotenuse is the longest side of the right triangle = x

Plug in values and solve for x.

We have:

`\begin{gathered} sin59=(400)/(x) \\ \\ x=(400)/(sin59) \\ \\ x=(400)/(0.8572) \\ \\ x=466.7\text{ ft} \end{gathered}`

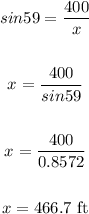
Therefore, the value of x is 466.7 ft.

ANSWER:

466.7 ft