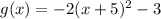Answer
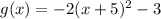
0. Horizontal translation 5 units.
,
1. Reflection over the x-axis
,
2. Vertical compression 2 units
,
3. Vertical translation down 3 units
Step-by-step explanation
• Writing the function in completed-square form.
As a ≠ 1, where a is the coefficient of the leading term, to write it in the completed-square form we have to make a = 1:
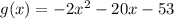
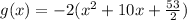
Now we have to take half of the x term and square it and add it to the function as follows:

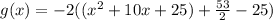
Finally, we have a Perfect Squared Trinomial in the left side that we can rewrite as follows, obtaining our function g(x):
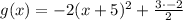
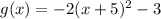
As our parent function is:

Then, the transformations that suffered were:
• Horizontal translation to the left 5 units
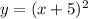
• Reflection over the x-axis
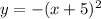
• Vertical compression 2 units
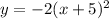
• Vertical translation down 3 units