Question

The number of bacteria in a certain sample increases according to the following function, where y0 is the initial number present, and y is the number present at t (in hours).y=y0e^0.067tHow many hours does it take for the size of the samples to double. Do not round any intermediate computations, and round your answer to the nearest tenths.

Answer 1

Answer: 10.3

Given:

`y=y_0e^(0.067t)`

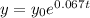
We are to find how many hours would it take for the size of the samples to double. Given that y is the number present at t hours, we know that we need to find how long would it take for y = 2y0.

We can now solve this by substituting y = 2y0

`y=y_0e^(0.067t)`
`2y_0=y_0e^(0.067t)`

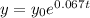
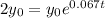
*cancel out y0

`2=e^(0.067t)`

*solve for t

`\ln 2=0.067t`
`t=(\ln 2)/(0.067)`
`t=10.345\approx10.3`

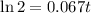
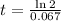
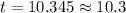
Therefore, at 10.3 hours, the sample will double in size.