Question

Complete the ordered pairs so they are solutions of the equation: 6x+y=12( , 0 )( 0 , )( , 6 )

Answer 1

Incomplete ordered pairs which are solutions to the equation 6x+y=12 are given.

Ordered pairs that are solutions to the given equation satisfy the equation.

To find the missing coordinate, substitute the known coordinate into the equation and solve for the missing one.

Recall that the first coordinate is the x-coordinate while the second is the y-coordinate.

The first incomplete ordered pair is ( ,0). So substitute y=0 and solve for x in the resulting equation:

`\begin{gathered} 6x+y=12;y=0 \\ \Rightarrow6x+0=12 \\ \Rightarrow6x=12 \\ \Rightarrow(6x)/(6)=(12)/(6) \\ \Rightarrow x=2 \end{gathered}`

Hence, the complete ordered pair is (2,0).

The second ordered pair is given as (0, ). So substitute x=0 into the equation and solve for y:

`\begin{gathered} 6x+y=12;x=0 \\ \Rightarrow6(0)+y=12 \\ \Rightarrow0+y=12 \\ \Rightarrow y=12 \end{gathered}`

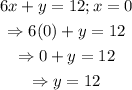
Hence, the complete ordered pair is (0,12).

The third ordered pair is ( ,6). So substitute y=6 into the equation and solve for x:

`\begin{gathered} 6x+y=12;y=6 \\ \Rightarrow6x+6=12 \\ \Rightarrow6x=12-6 \\ \Rightarrow6x=6 \\ \Rightarrow(6x)/(6)=(6)/(6) \\ \Rightarrow x=1 \end{gathered}`

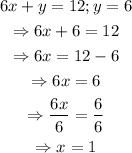
Hence, the complete ordered pair is (1,6).

The complete ordered pairs are: (2,0), (0,12), (1,6)