Question

Solve. ln(–x + 1) – ln(3x + 5) = ln(–6x + 1) –0.67 or 2–1.58 or 0.14–0.14 or 1.58–2 or 0.67

Answer 1

We have

`\ln \mleft(-x+1\mright)-ln\mleft(3x+5\mright)=ln\mleft(-6x+1\mright)`

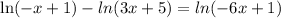
We need to simplify by applying logarithms rules

`\ln \mleft(-x+1\mright)=ln\mleft(-6x+1\mright)+ln\mleft(3x+5\mright)`
`ln(-x+1)=\ln ((-6x+1)(3x+5))`

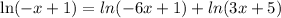
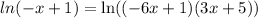
Then we apply the exponential on both sides

`-x+1=(-6x+1)(3x+5)`

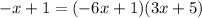
We simplify

`18x^2+26x-4=0`

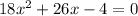
Then we solve the second equation degree with the general formula

`x_(1,2)=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}`

where

a=18

b=26

c=-4

we substitute the values

`x_(1,2)=\frac{-26\pm\sqrt[]{(26)^2-4(18)(-4)}}{2(18)}`

`x_(1,2)=\frac{-26\pm2\sqrt[]{241}}{36}`

We reduce and the solution is

`x_1=\frac{-13+\sqrt[]{241}}{18}=0.14`
`x_2=\frac{-13-\sqrt[]{241}}{18}=-1.58`

ANSWER

–1.58 or 0.14