Question

4. Graph the inequality:
`y \ \textless \ x ^2 + 2x - 3`

Answer 1

Step-by-step explanation:

First, we need to graph the equality y = x² + 2x - 3

So, to graph the parabola, we need to identify the vertex and 2 points in the parabola.

The vertex can be calculated as:

`x=-(b)/(2a)=-(2)/(2(1))=-1`

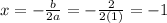
Where b is the number beside the x and a is the number beside the x². Then the value of y is equal to:

`\begin{gathered} y=x^2+2x-3 \\ y=(-1)^2+2(-1)-3 \\ y=1-2-3 \\ y=-4 \end{gathered}`

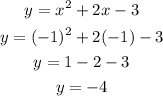
So, the vertex (- 1, - 4)

Then, to find 2 points in the parabola we can replace x by 0 and x by -2 as:

For x = 0

`\begin{gathered} y=x^2+2x-3 \\ y=0^2+2\cdot0-3 \\ y=-3 \end{gathered}`

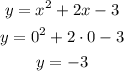
For x = -2

`\begin{gathered} y=(-2)^2+2\cdot(-2)-3 \\ y=4-4-3=-3 \end{gathered}`

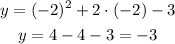
Therefore, we have the vertex (-1, -4) and the points (0, -3) and (-2, -3)

Then, the inequality is y less or equal than the parabola so, the zone for the inequality is:

So, the graph of the inequality is the region below the graph of the parabola.