Since it is a right triangle then you can use the Pythagorean theorem to find the measure of the segment DF, like this
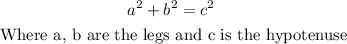
In this case, you have
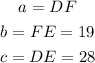
![\begin{gathered} a^2+b^2=c^2 \\ a^2+(19)^2=(28)^2 \\ \text{ Subtract (19)}^2\text{ on both sides of the equation} \\ a^2+(19)^2-(19)^2=(28)^2-(19)^2 \\ a^2=(28)^2-(19)^2 \\ \text{Apply square root on both sides of the equation} \\ \sqrt[]{a^2}=\sqrt[]{(28)^2-(19)^2} \\ a=20.57 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/c0v0ot03dlni3fbf0zxtos8xjtzqp6ys93.png)
Now to find the measure of angle D, you can use the trigonometric ratio
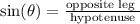
In this case, you have

Finally, to find the measure of angle E, you can use the trigonometric ratio
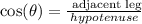
In this case, you have
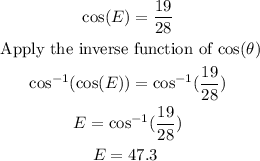
Therefore,
*The measure of the segment DF is 20.57.
*The measure of angle D is 42.7°.
*The measure of angle E is 47.3.