We have to find the minimum sample size.
The margin of error that it is aimed is ±0.2 hours from the real mean.
The population standard deviation is 0.8 hours.
The desired level of confidence is 96%. This corresponds to a z-score of 2.054.
We can relate sample size with the given information as:
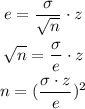
If we replace e = 0.2, σ = 0.8 and z = 2.054 we can calculate the sample size n as:
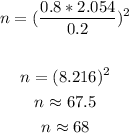
Answer: the sample size has to be at least 68 people.
W