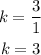Question

If dilating a shape maps it onto another shape, then the two shapes are similar. In this activity, you will use dilation to determine whether the shapes in these graphs are similar. Is there a dilation that maps shape 1 onto shape 11? If so, what is the scale factor and is it an enlargement or a reduction?

Answer 1

Dilations are transformations that change the shape if a determined figure, by enlarging the figure or reducing it.

Dilations involve multiplying the coordinates of the vertices of the figure by a sclale factor symbolized as "k" (or the lengths of the sides of the figure)

If k>0 → the figure gets enlarged.

If k<0 → the figure is reduced.

Shape I is smaller than shape II which means that the figure got enlarged.

To determine the scale factor you can determine the length of two corresponding sides, and divide the bigger one by the smaller one:

The scale factor is

`\begin{gathered} k=(3)/(1) \\ k=3 \end{gathered}`