toLet's begin by listing out the information given us:
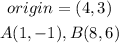
For a circle, we have the radius represented by r, the origin denoted by (h,k) with coordinates being (x, y)
To determine the equation of the line that goes through points A and B, we solve thus:
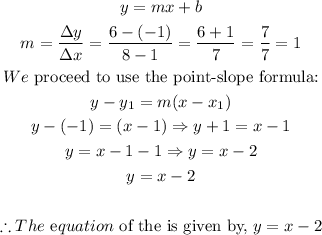
To determine if this line passes through the origin, we simply substitute the coordinate of the origin into the equation of the line:
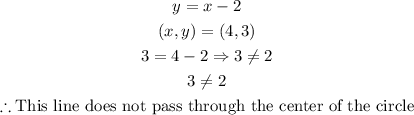
Therefore, this line does not pass through the center of the circle