Question

A student drops a ball from a height of 49.0m. If the ball increases speed at a uniform rate of 9.81m/s2, determine all unknowns. How long did the ball remain in the air? What was the ball’s speed just before striking the ground?

Answer 1

t = 3.16 s

v = 31 m/s

Step-by-step explanation

The ball is drop from the given height, so we can assume that the initial velocity of the ball was zero. Now, the initial height y0 was 49m and we can assume that the ground is the reference - i.e. final height is zero. Using the height formula:

`y=y_0+v_0t-(1)/(2)gt^2`

We have v0 = 0 and y = 0:

`0=49m-(1)/(2)\cdot9.81m/s^2_{}\cdot t^2`

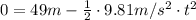
Solving for t:

`t=\sqrt[]{(2\cdot49m)/(9.81m/s^2)}=3.16s`

The ball was in the air of 3.16 seconds.

Now, with the speed formula:

`v=v_0+gt`

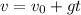
We've stated that v0 = 0, and we just found the time the ball was in the air:

`v=9.81m/s^2\cdot3.16s\approx31m/s`

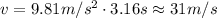
The speed of the ball just before strinking the ground was 31 m/s