Question

What is the axis of symmetry for the quadratic equation from Question 1: f(x) = 0.5(x+3)(x-7)?

Answer 1

Axis of symmetry of a quadratic equation

We know that for an equation of the form:

`f(x)=ax^2+bx+c`

the axis of symmetry is given by:

`x=-(b)/(2a)`

In this case, where our formula is given by the equation:

`f\mleft(x\mright)=0.5\mleft(x+3\mright)\mleft(x-7\mright)`

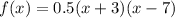
we want to multiply all the factors so we can work with the form of the first equation. We do this using the distributive property:

0.5 (x + 3) = 0.5 · x + 0.5 · 3

= 0.5x + 1.5

Then, in the equation:

`\begin{gathered} f(x)=0.5(x+3)(x-7) \\ \downarrow \\ f(x)=(0.5x+1.5)(x-7) \end{gathered}`

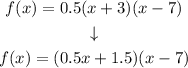
Now, multiplying both (0.5x + 1.5) and (x - 7) we have that:

`\begin{gathered} f(x)=(0.5x+1.5)(x-7) \\ \downarrow \\ f(x)=(0.5x+1.5)\cdot x-(0.5x+1.5)\cdot7 \end{gathered}`

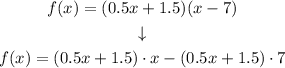
Now, finding the product of

(0.5x + 1.5)x, we have that:

`\begin{gathered} \mleft(0.5x+1.5\mright)x \\ =0.5x\cdot x+1.5\cdot x \\ =0.5x^2+1.5x \end{gathered}`

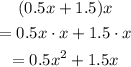
Replacing in the equation:

`\begin{gathered} f(x)=(0.5x+1.5)\cdot x-(0.5x+1.5)\cdot7 \\ \downarrow \\ f(x)=0.5x^2+1.5x-(0.5x+1.5)\cdot7 \end{gathered}`

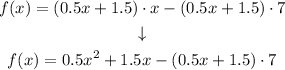
On the other hand, finding the product of

-(0.5x + 1.5) · 7,we have that

-(0.5x + 1.5) · 7 = -0.5x · 7 - 1.5 · 7

= -3.5x - 10.5

Replacing in the equation:

`\begin{gathered} f(x)=0.5x^2+1.5x-(0.5x+1.5)\cdot7 \\ \downarrow \\ f(x)=0.5x^2+1.5x-3.5x-10.5 \\ f(x)=0.5x^2-2x-10.5 \end{gathered}`

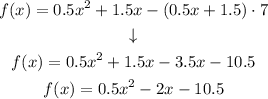
Then, we have that

a = 0.5,

b = -2

and

c = -10.5

Then, using the equation for the axis of symmetry, we have that:

`\begin{gathered} x=-(b)/(2a) \\ \downarrow \\ x=-((-2))/(2\cdot0.5) \\ x=(2)/(2\cdot0.5)=(1)/(0.5) \\ \downarrow \\ x=2 \end{gathered}`