Given a series, the ratio test implies finding the following limit:

If r<1 then the series converges, if r>1 the series diverges and if r=1 the test is inconclusive and we can't assure if the series converges or diverges. So let's see the terms in this limit:
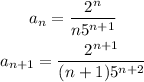
Then the limit is:

We can simplify the expressions inside the absolute value:
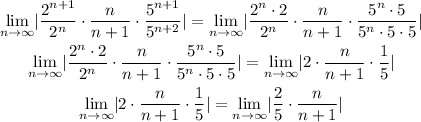
Since none of the terms inside the absolute value can be negative we can write this with out it:
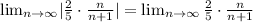
Now let's re-writte n/(n+1):
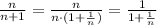
Then the limit we have to find is:
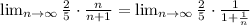
Note that the limit of 1/n when n tends to infinite is 0 so we get:
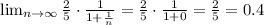
So from the test ratio r=0.4 and the series converges. Then the answer is the second option.